Comparision of material models: Steel01Thermal, Steel02Thermal and SteelECThermal using a restrained beam example
Developed by Domada Veera Venkata Ramakanth
Introduction
Figure-1 shows a restrained beam subjected to a temperature rise. The beam has been modelled using three existing steel models catering for thermal material degradation. The temperature load has been appl;ied on the left half portion of the beam. Displacement of the middle node and reaction at the right support have been compared in all three cases.
| OpenSees Model | Type used for this example |
|---|---|
| Element | DispBeamColumnThermal |
| Section | FiberSectionThermal |
| Material | Steel01Thermal, Steel02Thermal, SteelECThermal |
| Thermal Loading | Linear temperature rise over the left half of the member |
Download: This Example Package
Model Geometry
Tcl scripts for model definition
Restrained Beam model
wipe;
set s1 1;
set s2 2;
set s3 3;
model BasicBuilder -ndm 2 -ndf 3
# node $NodeTag $XCoord $Ycoord
node 1 2000 0
node 2 1000 0
node 3 0 0
# fix $NodeTag x-transl y-transl z-rot
fix 1 1 1 1
fix 3 1 1 1
# Geometric Transformation
geomTransf Linear 1
geomTransf PDelta 2
geomTransf Corotational 3
#uniaxialMaterial Steel01Thermal $matTag $Fy $E0 $b <$a1 $a2 $a3 $a4>
uniaxialMaterial Steel01Thermal 1 500 2e+05 0.15
#uniaxialMaterial Steel02Thermal $matTag $Fy $E0 $b $R0 $cR1 $cR2 <$a1 $a1 $a1 $a1>
uniaxialMaterial Steel02Thermal 2 500 2e+05 0.15 20 0.925 0.15 0 1 0 1 0
#uniaxialMaterial SteelECThermal $matTag <$steelType> $Fy $E0
uniaxialMaterial SteelECThermal 3 500 2e+05
section fiberSecThermal 155 -GJ 10000 {
#patch quad $matTag $numSubdivIJ $numSubdivJK $yI $zI $yJ $zJ $yK $zK $yL $zL
patch quad $s1 10 10 -50.0 -50.0 50.0 -50.0 50.0 50.0 -50.0 50.0
}
# element dispBeamColumn $eleTag $iNode $jNode $numIntgrPts $secTag $transfTag
element dispBeamColumnThermal 1 3 2 3 155 1 -mass 0
element dispBeamColumnThermal 2 2 1 3 155 1 -mass 0
recorder Node -file Node_displacements-s1.out -node 2 -dof 1 disp
# recorder Node -file Node_rotations.out -time -nodeRange 1 3 -dof 3 disp
recorder Node -file Node_forceReactions-s1.out -node 3 -dof 1 reaction
# recorder Node -file Node_momentReactions.out -time -nodeRange 1 3 -dof 3 reaction
# recorder Element -file DispBeamColumn_localForce.out -time -ele 1 2 localForce
# recorder Element -file DispBeamColumn_basicDeformation.out -time -ele 1 2 basicDeformation
# recorder Element -file DispBeamColumn_plasticDeformation.out -time -ele 1 2 plasticDeformation
puts "Running interval 1\n"
# Loads - Plain Pattern
pattern Plain 100 Linear {
eleLoad -ele 1 -type -beamThermal 1000 -50 1000 50.0
}
# recording the initial status
record
# Analysis options
system BandGeneral
numberer Plain
constraints Transformation
integrator LoadControl 0.01
test NormDispIncr 0.001 50 2
algorithm Newton
analysis Static
analyze 100
Output Results
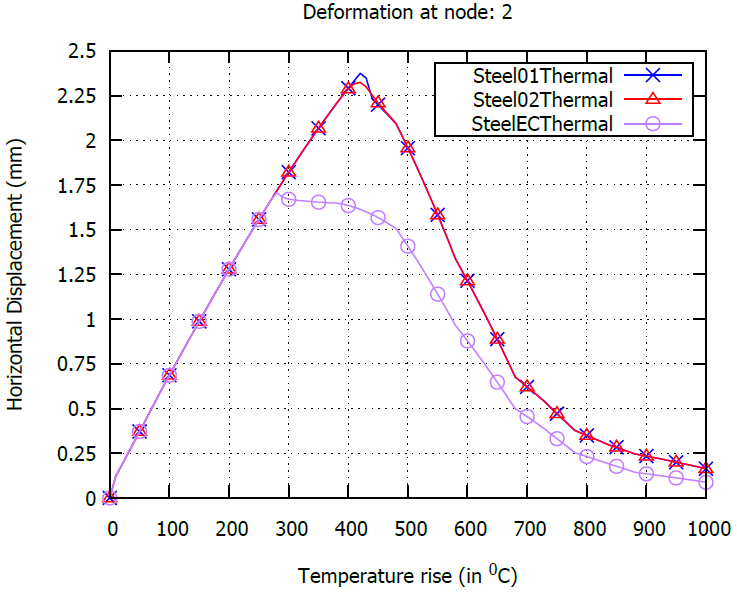
Comparision of horizontal displacement of the middle node
Comparision of reaction force at the right side support

Closed form solution
The solution can be verified using a closed form expression as shown given below.
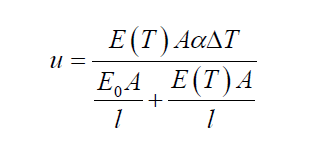
Here u is the horizontal displacement of middle node, Eo and E(T) are the elastic modulous at ambient and elevated temperature respectively
This page is created by D.V.V. Ramakanth, 2020